7.4 The other trigonometric functions (Page 3/14)

Page 3 / 14

Try It

Use reference angles to find all six trigonometric functions of $- \frac{7 π}{4} .$

$\begin{array}{l} \sin (\frac{- 7 π}{4}) = \frac{\sqrt{2}}{2}, \cos (\frac{- 7 π}{4}) = \frac{\sqrt{2}}{2}, \tan (\frac{- 7 π}{4}) = 1, \\ \sec (\frac{- 7 π}{4}) = \sqrt{2}, \csc (\frac{- 7 π}{4}) = \sqrt{2}, \cot (\frac{- 7 π}{4}) = 1 \end{array}$

Got questions? Get instant answers now!

Using even and odd trigonometric functions

To be able to use our six trigonometric functions freely with both positive and negative angle inputs, we should examine how each function treats a negative input. As it turns out, there is an important difference among the functions in this regard.

Consider the function $f (x) = x^{2},$ shown in [link] . The graph of the function is symmetrical about the y -axis. All along the curve, any two points with opposite x -values have the same function value. This matches the result of calculation: ${(4)}^{2} = {(−4)}^{2}, {(−5)}^{2} = {(5)}^{2},$ and so on. So $f (x) = x^{2}$ is an even function, a function such that two inputs that are opposites have the same output. That means $f (- x) = f (x) .$

This is an image of a graph of and upward facing parabola with points (-2, 4) and (2, 4) labeled. — The function $f (x) = x^{2}$ is an even function.

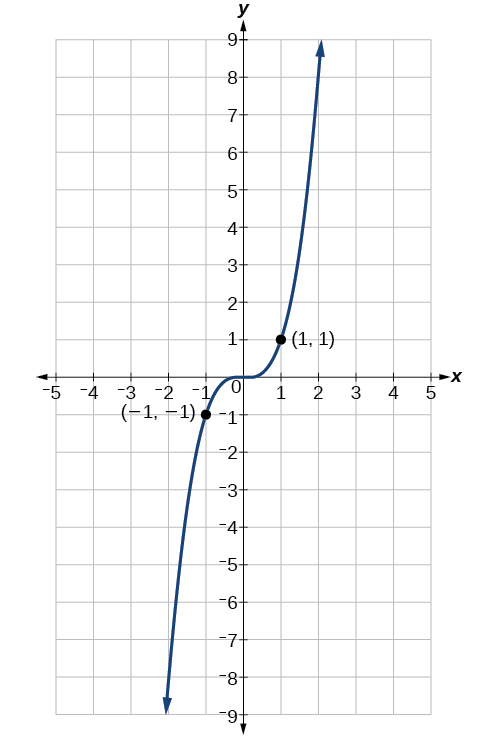
Now consider the function $f (x) = x^{3},$ shown in [link] . The graph is not symmetrical about the y -axis. All along the graph, any two points with opposite x -values also have opposite y -values. So $f (x) = x^{3}$ is an odd function, one such that two inputs that are opposites have outputs that are also opposites. That means $f (- x) = - f (x) .$

This is an image of a graph of the function f of x = x to the third power with labels for points (-1, -1) and (1, 1). — The function $f (x) = x^{3}$ is an odd function.

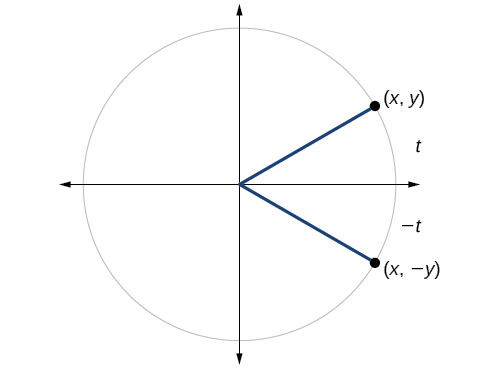
We can test whether a trigonometric function is even or odd by drawing a unit circle with a positive and a negative angle, as in [link] . The sine of the positive angle is $y .$ The sine of the negative angle is $- y .$ The sine function, then, is an odd function. We can test each of the six trigonometric functions in this fashion. The results are shown in [link] .

Graph of circle with angle of t and -t inscribed. Point of (x, y) is at intersection of terminal side of angle t and edge of circle. Point of (x, -y) is at intersection of terminal side of angle -t and edge of circle.

$\begin{matrix} sin t & = & y \\ sin (- t) & = & - y \\ sin t & \neq & sin (- t) \end{matrix}$	$\begin{matrix} cos t & = & x \\ cos (- t) & = & x \\ cos t & = & cos (- t) \end{matrix}$	$\begin{matrix} tan (t) & = & \frac{y}{x} \\ tan (- t) & = & - \frac{y}{x} \\ tan t & \neq & tan (- t) \end{matrix}$
$\begin{matrix} sec t & = & \frac{1}{x} \\ sec (- t) & = & \frac{1}{x} \\ sec t & = & sec (- t) \end{matrix}$	$\begin{matrix} csc t & = & \frac{1}{y} \\ csc (- t) & = & \frac{1}{- y} \\ csc t & \neq & csc (- t) \end{matrix}$	$\begin{matrix} cot t & = & \frac{x}{y} \\ cot (- t) & = & \frac{x}{- y} \\ cot t & \neq & cot (- t) \end{matrix}$

A General Note

Even and odd trigonometric functions

An even function is one in which $f (- x) = f (x) .$

An odd function is one in which $f (- x) = - f (x) .$

Cosine and secant are even:

\begin{matrix} \cos (- t) & = & cos t \\ \sec (- t) & = & sec t \end{matrix}

Sine, tangent, cosecant, and cotangent are odd:

\begin{matrix} sin (- t) & = & - sin t \\ tan (- t) & = & - tan t \\ csc (- t) & = & - csc t \\ cot (- t) & = & - cot t \end{matrix}

Using even and odd properties of trigonometric functions

If the secant of angle $t$ is 2, what is the secant of $- t ?$

Secant is an even function. The secant of an angle is the same as the secant of its opposite. So if the secant of angle $t$ is 2, the secant of $- t$ is also 2.

Got questions? Get instant answers now!

Try It

If the cotangent of angle $t$ is $\sqrt{3},$ what is the cotangent of $- t ?$

$- \sqrt{3}$

Got questions? Get instant answers now!

Recognizing and using fundamental identities

We have explored a number of properties of trigonometric functions. Now, we can take the relationships a step further, and derive some fundamental identities. Identities are statements that are true for all values of the input on which they are defined. Usually, identities can be derived from definitions and relationships we already know. For example, the Pythagorean Identity we learned earlier was derived from the Pythagorean Theorem and the definitions of sine and cosine.

A General Noter

Fundamental identities

We can derive some useful identities from the six trigonometric functions. The other four trigonometric functions can be related back to the sine and cosine functions using these basic relationships:

\tan t = \frac{\sin t}{\cos t}

\sec t = \frac{1}{\cos t}

\csc t = \frac{1}{\sin t}

cot t = \frac{1}{tan t} = \frac{cos t}{sin t}

<< Chapter < Page Page > Chapter >>

Practice Key Terms 6

Read also:

Get Jobilize Job Search Mobile App in your pocket Now!

100% Free Mobile Applications
Receive real-time job alerts and never miss the right job again

Source: OpenStax, Algebra and trigonometry. OpenStax CNX. Nov 14, 2016 Download for free at https://legacy.cnx.org/content/col11758/1.6

Google Play and the Google Play logo are trademarks of Google Inc.

Notification Switch

Would you like to follow the 'Algebra and trigonometry' conversation and receive update notifications?

Ask

	5 Alternate forms of the pythagorean identity By OpenStax Read Online Course
	3 Using even and odd trigonometric functions By OpenStax Read Online Course
	Tournament Director Quiz By Eddie Unverzagt Start Quiz
	12 Dr Dowers Endocrinology By Brooke Delaney Start Exam
	Spanish (Places) By Inderjeet Brar Start Flashcards
	1 Biology 01 The Study of Life MCQ By OpenStax Start Quiz
	6 AP Key Terms 06 The Skeletal System By OpenStax Start Key Terms
	1 Understanding Societies 1 By Jessica Collett Start Exam
	23 Biology 23 Protists MCQ By OpenStax Start Quiz
	20 AP 20 Blood Vessels Circulation Essay By OpenStax Start Flashcards
	25 AP Key Terms 25 The Urinary System By OpenStax Start Key Terms
	Nutrition Exam By Hannah Sheth Start Quiz