11.3 Systems of nonlinear equations and inequalities: two variables  (Page 2/9)

Intersection of a circle and a line

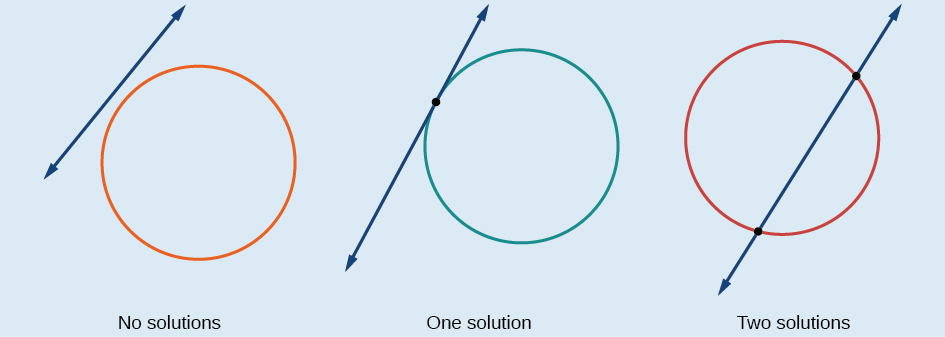
Just as with a parabola and a line, there are three possible outcomes when solving a system of equations representing a circle and a line.

A General Note

Possible types of solutions for the points of intersection of a circle and a line

[link] illustrates possible solution sets for a system of equations involving a circle and a line.

• No solution. The line does not intersect the circle.
• One solution. The line is tangent to the circle and intersects the circle at exactly one point.
• Two solutions. The line crosses the circle and intersects it at two points.

Finding the intersection of a circle and a line by substitution

Find the intersection of the given circle and the given line by substitution.

$$\begin{array}{l}{x}^2+y^2=5\hfill \\ y=3x\mathrm{-5}\hfill \end{array}$$

One of the equations has already been solved for $y.$ We will substitute $y=3x\mathrm{-5}$ into the equation for the circle.

$$\begin{array}{c}{x}^2+{(3x\mathrm{-5})}^2=5\\ x^2+9x^2\mathrm{-30}x+25=5\\ 10x^2\mathrm{-30}x+20=0\end{array}$$

Now, we factor and solve for $x.$

$$\begin{array}{l}10(x^2-3x+2)=0\hfill \\ 10(x-2)(x-1)=0\hfill \\ x=2\hfill \\ x=1\hfill \end{array}$$

Substitute the two x -values into the original linear equation to solve for $y.$

$$\begin{array}{l}y=3(2)\mathrm{-5}\hfill \\ =1\hfill \\ \hfill \\ \hfill \\ \hfill \\ y=3(1)\mathrm{-5}\hfill \\ =\mathrm{-2}\hfill \end{array}$$

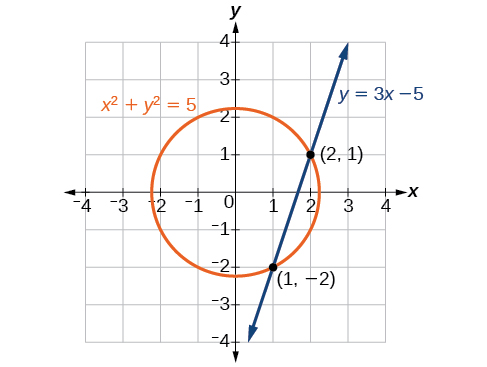
The line intersects the circle at $\left(2,1\right)$ and $\left(1,\mathrm{-2}\right),$ which can be verified by substituting these $\left(x,y\right)$ values into both of the original equations. See [link] .

Got questions? Get instant answers now!

Got questions? Get instant answers now!

Solving a system of nonlinear equations using elimination

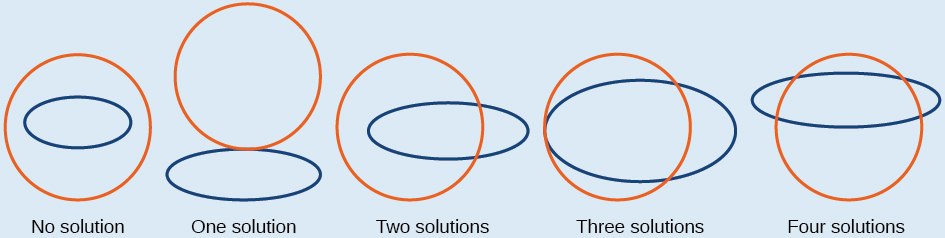
We have seen that substitution is often the preferred method when a system of equations includes a linear equation and a nonlinear equation. However, when both equations in the system have like variables of the second degree, solving them using elimination by addition is often easier than substitution. Generally, elimination is a far simpler method when the system involves only two equations in two variables (a two-by-two system), rather than a three-by-three system, as there are fewer steps. As an example, we will investigate the possible types of solutions when solving a system of equations representing a circle and an ellipse.

A General Note

Possible types of solutions for the points of intersection of a circle and an ellipse

[link] illustrates possible solution sets for a system of equations involving a circle and an ellipse .

• No solution. The circle and ellipse do not intersect. One shape is inside the other or the circle and the ellipse are a distance away from the other.
• One solution. The circle and ellipse are tangent to each other, and intersect at exactly one point.
• Two solutions. The circle and the ellipse intersect at two points.
• Three solutions. The circle and the ellipse intersect at three points.
• Four solutions. The circle and the ellipse intersect at four points.