| << Chapter < Page | Chapter >> Page > |
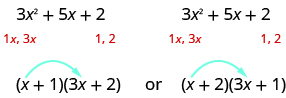
Which factors are correct? To decide that, we multiply the inner and outer terms.

Since the middle term of the trinomial is 5 x , the factors in the first case will work. Let’s FOIL to check.
Our result of the factoring is:
When the middle term is negative and the last term is positive, the signs in the binomials must both be negative.
Factor completely: .
| The trinomial is already in descending order. |
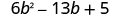 |
| Find the factors of the first term. |
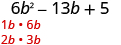 |
| Find the factors of the last term. Consider the signs. Since the last term, 5 is positive its factors must both be positive or both be negative. The coefficient of the middle term is negative, so we use the negative factors. |
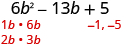 |
Consider all the combinations of factors.
| Possible factors | Product |
|---|---|
| * | |
When we factor an expression, we always look for a greatest common factor first. If the expression does not have a greatest common factor, there cannot be one in its factors either. This may help us eliminate some of the possible factor combinations.
Factor completely: .
| The trinomial is already in descending order. |
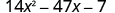 |
| Find the factors of the first term. |
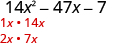 |
| Find the factors of the last term. Consider the signs. Since it is negative, one factor must be positive and one negative. |
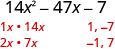 |
Consider all the combinations of factors. We use each pair of the factors of with each pair of factors of
| Factors of | Pair with | Factors of |
|---|---|---|
| , |
,
, (reverse order) | |
| , |
,
, (reverse order) | |
|
,
, (reverse order) | ||
|
,
, (reverse order) |
These pairings lead to the following eight combinations.

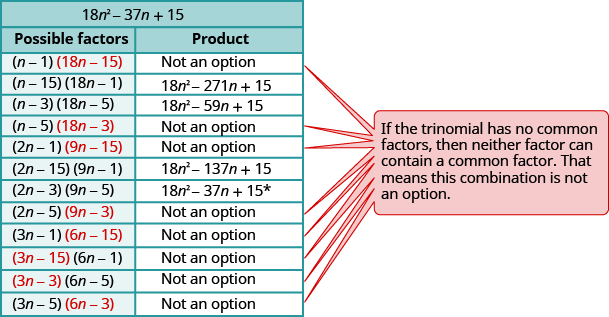
Factor completely: .
| The trinomial is already in descending order. | |
| Find the factors of the first term. |
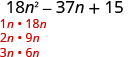 |
| Find the factors of the last term. Consider the signs. Since 15 is positive and the coefficient of the middle term is negative, we use the negative facotrs. |
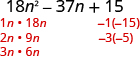 |
Consider all the combinations of factors.

Notification Switch
Would you like to follow the 'Elementary algebra' conversation and receive update notifications?