| << Chapter < Page | Chapter >> Page > |
The trigonometric form of the Fourier Series, shown in [link] can be converted into a more convenient form by doing the following substitutions:
After some straight-forward rearranging, we obtain
Keeping in mind that and are only defined for positive values of , lets sum over the negative integers in the second summation:
Next, let's assume that and are defined for both positive and negative . In this case, we find that and , since
and
Using this fact, we can rewrite [link] as
If we define The notation “ " is often used instead of the “ " sign when defining a new variable. , and
then we can rewrite [link] as
which is called the complex form of the Fourier Series. Note that since and , we have
This means that
and
Next, we must find formulas for finding the given . We first look at a property of complex exponentials:
To see this, we note that
It's easy to see that also has period , hence the integral is over periods of and . Therefore, if , then
otherwise
We use [link] to derive an equation for as follows. Consider the integral
Substituting the complex form of the Fourier Series of in [link] , (using as the index of summation) we obtain
Rearranging the order of integration and summation, combining the exponents, and using [link] gives
Using this result, we find that
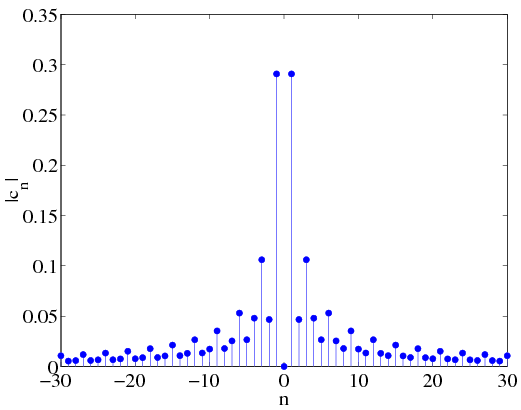
Example 2.1 Let's now find the complex form of the Fourier Series for the signal in Example [link] . The integral to be evaluated is
Integrating by parts yields
[link] shows the magnitude of the coefficients, . Note that the complex Fourier Series coefficients have even symmetry as was mentioned earlier.
Can the basic formula for computing the in [link] be simplified when has either even, odd, or half-wave symmetry? The answer is yes. We simply use the fact that
and solve for and using the formulae given above for even, odd, or half-wave symmetric signals. This avoids having to integrate complex quantities. This can also be seen by noting that (setting in [link] ):
Alternately, if has half-wave symmetry, we can use [link] , [link] , and [link] to get
Unlike the trigonometric form, we cannot simplify this further if is even or odd symmetric since has neither even nor odd symmetry.
Example 2.2 In this example we will look at the effect of adjusting the period of a pulse train signal. Consider the signal depicted in [link] .
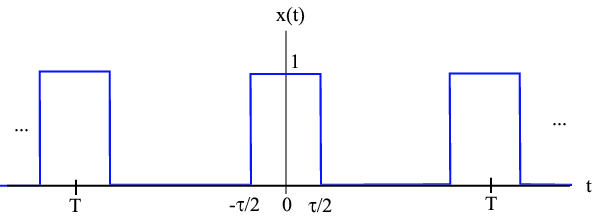
The Fourier Series coefficients for this signal are given by
[link] shows the magnitude of , the amplitude spectrum , for and as well as the Fourier Series for the signal based on the first 30 coefficients
Similar plots are shown in Figures [link] , and [link] , for , and , respectively.
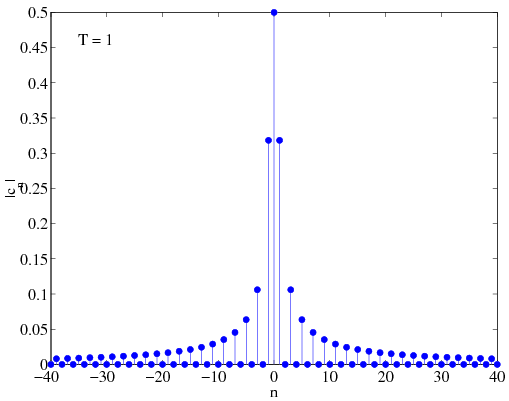

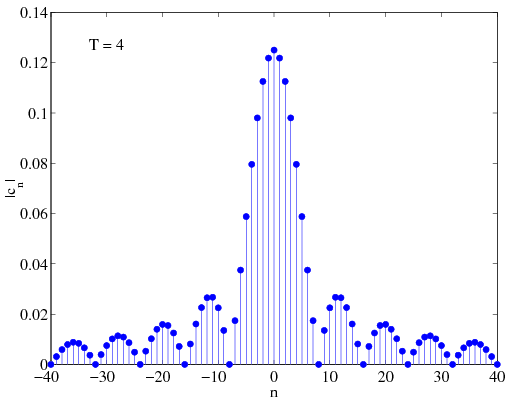
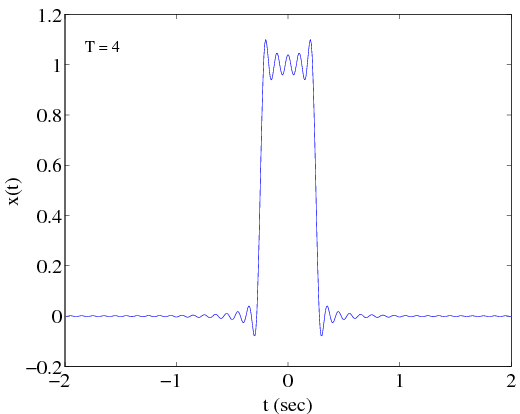
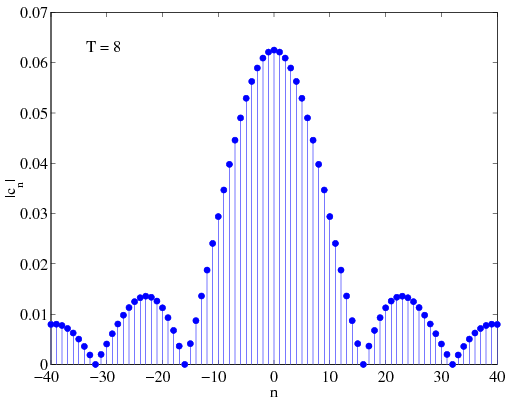
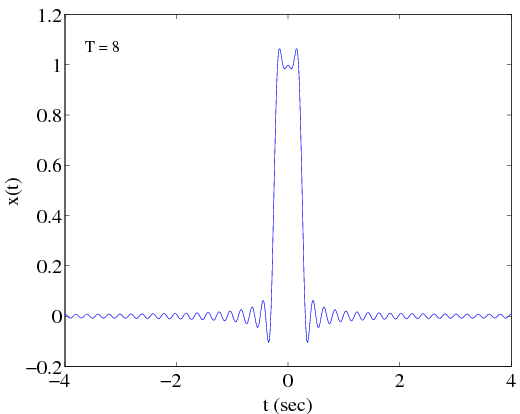
This example illustrates several important points about the Fourier Series: As the period increases, decreases in magnitude (this is obvious since ). Therefore, as the period increases, successive Fourier Series coefficients represent more closely spaced frequencies. The frequencies corresponding to each are given by the following table:
| 0 | 0 |
This table establishes a relation between and the frequency variable . In particular, if , we have and
| 0 | 0 |
If , then and
| 0 | 0 |
and if , we have and
| 0 | 0 |
Note that in all three cases, the first zero coefficient corresponds to the value of for which . Also, as gets bigger, the appear to resemble more closely spaced samples of a continuous function of frequency (since the are more closely spaced). Can you determine what this function is?
As we shall see, by letting the period get large (infinitely large), we will derive the Fourier Transform in the next chapter.

Notification Switch
Would you like to follow the 'Signals, systems, and society' conversation and receive update notifications?