12.3 Continuity  (Page 2/10)

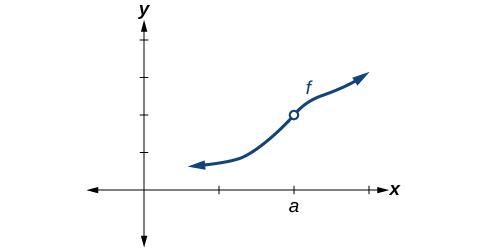
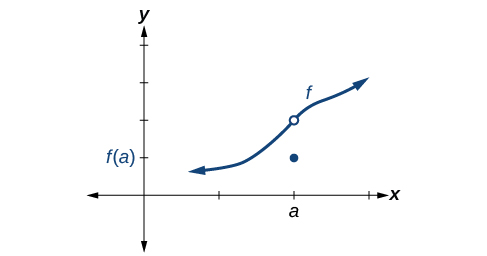
So how can we decide if a function is continuous at a particular number? We can check three different conditions. Let’s use the function $y=f\left(x\right)$ represented in [link] as an example.

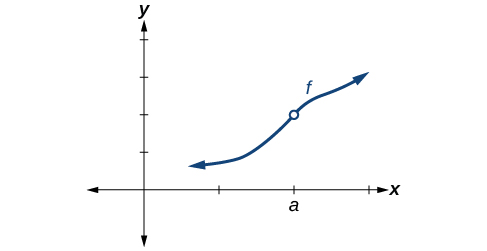
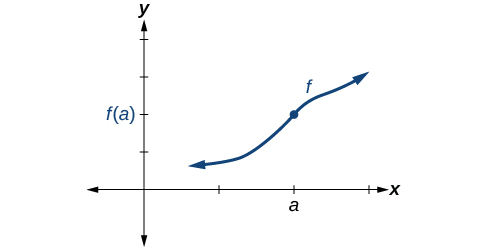
Condition 1 According to Condition 1, the function $f\left(a\right)$ defined at $x=a$ must exist. In other words, there is a y -coordinate at $x=a$ as in [link] .

Condition 2 According to Condition 2, at $x=a$ the limit, written $\underset{x\to a}{\lim }f(x),$ must exist. This means that at $x=a$ the left-hand limit must equal the right-hand limit. Notice as the graph of $f$ in [link] approaches $x=a$ from the left and right, the same y -coordinate is approached. Therefore, Condition 2 is satisfied. However, there could still be a hole in the graph at $x=a$ .

Condition 3 According to Condition 3, the corresponding $y$ coordinate at $x=a$ fills in the hole in the graph of $f.$ This is written $\underset{x\to a}{\lim }f(x)=f(a).$

Satisfying all three conditions means that the function is continuous. All three conditions are satisfied for the function represented in [link] so the function is continuous as $x=a.$

[link] through [link] provide several examples of graphs of functions that are not continuous at $x=a$ and the condition or conditions that fail.

Identifying a jump discontinuity

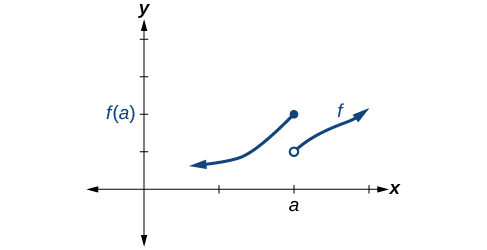
Discontinuity can occur in different ways. We saw in the previous section that a function could have a left-hand limit    and a right-hand limit    even if they are not equal. If the left- and right-hand limits exist but are different, the graph “jumps” at $x=a$ . The function is said to have a jump discontinuity.

As an example, look at the graph of the function $y=f\left(x\right)$ in [link] . Notice as $x$ approaches $a$ how the output approaches different values from the left and from the right.

Identifying removable discontinuity

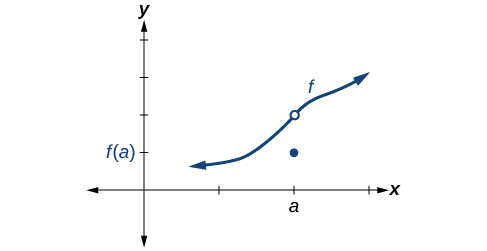
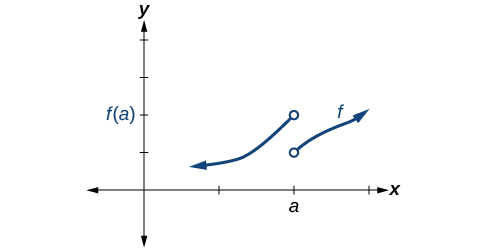
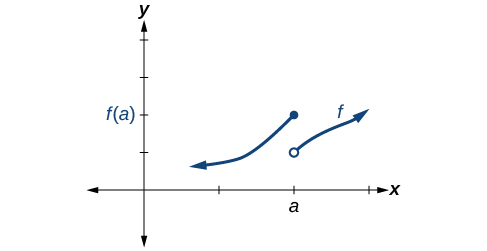
Some functions have a discontinuity, but it is possible to redefine the function at that point to make it continuous. This type of function is said to have a removable discontinuity. Let’s look at the function $y=f\left(x\right)$ represented by the graph in [link] . The function has a limit. However, there is a hole at $x=a$ . The hole can be filled by extending the domain to include the input $x=a$ and defining the corresponding output of the function at that value as the limit of the function at $x=a$ .