| << Chapter < Page | Chapter >> Page > |
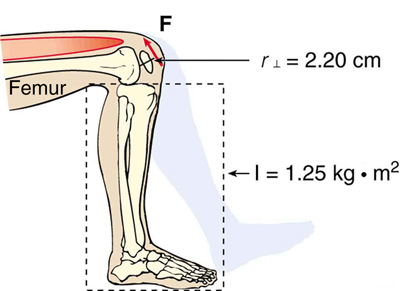
The person whose leg is shown in [link] kicks his leg by exerting a 2000-N force with his upper leg muscle. The effective perpendicular lever arm is 2.20 cm. Given the moment of inertia of the lower leg is , (a) find the angular acceleration of the leg. (b) Neglecting the gravitational force, what is the rotational kinetic energy of the leg after it has rotated through (1.00 rad)?
Strategy
The angular acceleration can be found using the rotational analog to Newton’s second law, or . The moment of inertia is given and the torque can be found easily from the given force and perpendicular lever arm. Once the angular acceleration is known, the final angular velocity and rotational kinetic energy can be calculated.
Solution to (a)
From the rotational analog to Newton’s second law, the angular acceleration is
Because the force and the perpendicular lever arm are given and the leg is vertical so that its weight does not create a torque, the net torque is thus
Substituting this value for the torque and the given value for the moment of inertia into the expression for gives
Solution to (b)
The final angular velocity can be calculated from the kinematic expression
or
because the initial angular velocity is zero. The kinetic energy of rotation is
so it is most convenient to use the value of just found and the given value for the moment of inertia. The kinetic energy is then
Discussion
These values are reasonable for a person kicking his leg starting from the position shown. The weight of the leg can be neglected in part (a) because it exerts no torque when the center of gravity of the lower leg is directly beneath the pivot in the knee. In part (b), the force exerted by the upper leg is so large that its torque is much greater than that created by the weight of the lower leg as it rotates. The rotational kinetic energy given to the lower leg is enough that it could give a ball a significant velocity by transferring some of this energy in a kick.
Angular momentum, like energy and linear momentum, is conserved. This universally applicable law is another sign of underlying unity in physical laws. Angular momentum is conserved when net external torque is zero, just as linear momentum is conserved when the net external force is zero.
We can now understand why Earth keeps on spinning. As we saw in the previous example, . This equation means that, to change angular momentum, a torque must act over some period of time. Because Earth has a large angular momentum, a large torque acting over a long time is needed to change its rate of spin. So what external torques are there? Tidal friction exerts torque that is slowing Earth’s rotation, but tens of millions of years must pass before the change is very significant. Recent research indicates the length of the day was 18 h some 900 million years ago. Only the tides exert significant retarding torques on Earth, and so it will continue to spin, although ever more slowly, for many billions of years.

Notification Switch
Would you like to follow the 'College physics' conversation and receive update notifications?