| << Chapter < Page | Chapter >> Page > |
Rigid bodies are composed of very small particles which interact with each other via electromagnetic force. They form a continuous distribution of mass. As such, expressions of COM in three coordinate directions involve evaluation of integrals as described in earlier module. This evaluation, however, is rendered difficult on two counts :
The geometry of regularly shaped bodies are defined by mathematical equations. Such is not the case with irregular bodies. However, there is a good thing about center of mass (COM) that it represents the point where external force equivalently applies. This fact allows us to experimentally determine COM of even irregularly shaped bodies. We can balance a body on a pointed wedge. The COM of the body falls on the line of balance. In order to know the COM (a point), however, we need to balance the body with different orientation to get another line of balance. The point of intersection of the two lines of balance is the COM of the body.
We are saved from any mathematical calculation in cases of certain regularly shaped bodies with uniform density, which are symmetric to all the axes of the coordinate system involved. In all such cases, COM is same as geometric center. COMs of a sphere, spherical shell, ring, disc, cylinder, cone, rod, square plate etc. fall under this category where COM is simply the geometric center of the bodies.
However, there are cases of regular shaped bodies which are not symmetric to three (for three dimensional bodies) or two axes (for planar bodies). For example, consider the case of hemispherical body or the case of semi-circular wire. These bodies are not symmetric to all the axes involved. Here, geometric center and, thus, COM are not obvious. In this section, we shall evaluate COM of such regular shaped bodies, which are not symmetric about all axes of the coordinate system.
Regular bodies allow us to evaluate integrals as geometry is defined. Evaluation of integral is simplified if the mass is evenly distributed. Unless otherwise indicated, we shall consider rigid bodies of uniform density only. Further, some rigid bodies are combination of other regular bodies, whose COMs are known. In that case, we would employ the formula of COM for the system of particles.
In this case, we need not resort to integration as L-shaped rod is simply a combination of two rods with known COMs, which can be treated as combination of particles.
L shaped rod
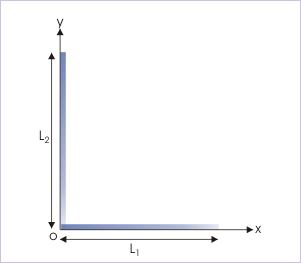
Let the origin of the planar coordinate system coincides with the corner of the L-shaped rod. COM of a uniform rod is the middle point of the rod. From the figure, the COM of rod along x-axis is ( ). On the other hand, COM of rod along y-axis is( ). These rods, therefore, can be considered as particles at these positions. Thus, the L-shaped rod system reduces to the case of two particles system separated by a distance. Let and be the mass of two rods respectively. Now, the linear mass density is :

Notification Switch
Would you like to follow the 'Physics for k-12' conversation and receive update notifications?