10.1 Angular acceleration  (Page 2/6)

If the bicycle in the preceding example had been on its wheels instead of upside-down, it would first have accelerated along the ground and then come to a stop. This connection between circular motion and linear motion needs to be explored. For example, it would be useful to know how linear and angular acceleration are related. In circular motion, linear acceleration is tangent to the circle at the point of interest, as seen in [link] . Thus, linear acceleration is called tangential acceleration     $a_{\text{t}}$ .

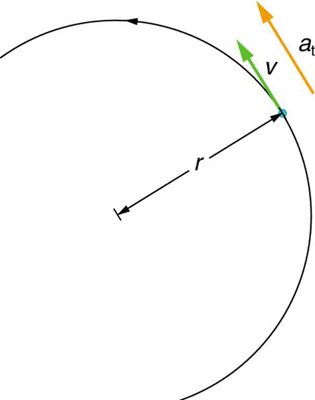
Linear or tangential acceleration refers to changes in the magnitude of velocity but not its direction. We know from Uniform Circular Motion and Gravitation that in circular motion centripetal acceleration, $a_{\text{c}}$ , refers to changes in the direction of the velocity but not its magnitude. An object undergoing circular motion experiences centripetal acceleration, as seen in [link] . Thus, $a_{\text{t}}$ and $a_{\text{c}}$ are perpendicular and independent of one another. Tangential acceleration $a_{\text{t}}$ is directly related to the angular acceleration $\alpha$ and is linked to an increase or decrease in the velocity, but not its direction.

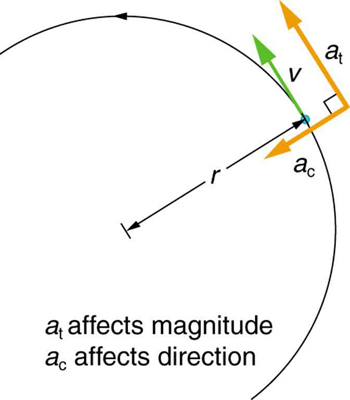
Now we can find the exact relationship between linear acceleration $a_{\text{t}}$ and angular acceleration $\alpha$ . Because linear acceleration is proportional to a change in the magnitude of the velocity, it is defined (as it was in One-Dimensional Kinematics ) to be

For circular motion, note that $v=\mathrm{r\omega }$ , so that

The radius $r$ is constant for circular motion, and so $\text{Δ}(\mathrm{r\omega })=r(\mathrm{\Delta }\omega )$ . Thus,

By definition, $\alpha =\frac{\mathrm{\Delta }\omega }{\mathrm{\Delta }t}$ . Thus,

or

These equations mean that linear acceleration and angular acceleration are directly proportional. The greater the angular acceleration is, the larger the linear (tangential) acceleration is, and vice versa. For example, the greater the angular acceleration of a car’s drive wheels, the greater the acceleration of the car. The radius also matters. For example, the smaller a wheel, the smaller its linear acceleration for a given angular acceleration $\alpha$ .

Calculating the angular acceleration of a motorcycle wheel

A powerful motorcycle can accelerate from 0 to 30.0 m/s (about 108 km/h) in 4.20 s. What is the angular acceleration of its 0.320-m-radius wheels? (See [link] .)

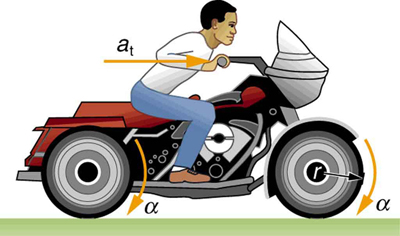
Strategy

We are given information about the linear velocities of the motorcycle. Thus, we can find its linear acceleration $a_{\text{t}}$ . Then, the expression $\alpha =\frac{a_{\text{t}}}{r}$ can be used to find the angular acceleration.

Solution

The linear acceleration is

$\begin{array}{lll}{a}_{\text{t}}& =& \frac{\mathrm{\Delta }v}{\mathrm{\Delta }t}\\ & =& \frac{\text{30.0 m/s}}{\text{4.20 s}}\\ & =& \text{7.14}{\text{m/s}}^2.\end{array}$

We also know the radius of the wheels. Entering the values for $a_{\text{t}}$ and $r$ into $\alpha =\frac{a_{\text{t}}}{r}$ , we get

$\begin{array}{lll}\alpha & =& \frac{a_{\text{t}}}{r}\\ & =& \frac{\text{7.14}{\text{m/s}}^2}{\text{0.320 m}}\\ & =& \text{22.3}{\text{rad/s}}^2.\end{array}$

Discussion

Units of radians are dimensionless and appear in any relationship between angular and linear quantities.

Got questions? Get instant answers now!