| << Chapter < Page | Chapter >> Page > |
Hence, domain of the given function is . The representation of the domain on real number line is shown with a dark line on either side of the excluded point “-1”.
Domain of a function

Problem 2 : A function is given by :
Determine its domain set.
Solution : We observe that given function is a square root of a quadratic polynomial. The expression within square root should be a non-negative number as square root of a negative number is not a real number. It means that expression under even root should be non-negative.
We factorize the quadratic expression in order to find corresponding interval for which expression under the root is non-negative.
We interpret this result in reference to quadratic equation. When x ≤ 2, both factors of quadratic equation are non-positive and their product is non-negative. We can check with one such value like “1” or “-1”. On the other hand, when x ≥ 3, both factors are non-negative and their product is also non-negative. It turns out that these two conditions correspond to two intervals, which are disconnected to each other.
Domain of a function
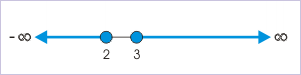
The representation of the domain interval on real number line is shown with thick line and small filled circles. From the representation on the figure also, it is clear that it is a case of two disjoint intervals. We, therefore, represent the valid domain of the function with the help of the concept of union of two sets (intervals) in the following manner :
i.e.
This interpretation is typical of product of two linear factors, which is greater than or equal to zero. This interpretation, as a matter of fact, can be used as an axiom in general for deciding interval, involving product of two factors.
Range is a set of images. It is a subset of co-domain. The requirement, here, is to find the interval of the co-domain for which there is “pre-image” in the domain set. In other words, we need to find the values of “y” within the domain of the function.
Further, we have already developed technique to find the inverse element i.e. pre-images, while studying inverse function. We shall apply the same concept here to decide range of the function. However, unlike determining domain, it is extremely helpful that we follow a step-wise algorithm to determine the range. It is given here as :

Notification Switch
Would you like to follow the 'Functions' conversation and receive update notifications?