This module is from Elementary Algebra by Denny Burzynski and Wade Ellis, Jr.
Beginning with the graphical solution of systems, this chapter includes an interpretation of independent, inconsistent, and dependent systems and examples to illustrate the applications for these systems. The substitution method and the addition method of solving a system by elimination are explained, noting when to use each method. The five-step method is again used to illustrate the solutions of value and rate problems (coin and mixture problems), using drawings that correspond to the actual situation.Objectives of this module: be able to recognize a system of equations and a solution to it, be able to graphically interpret independent, inconsistent, and dependent systems, be able to solve a system of linear equations graphically.
Overview
- Systems of Equations
- Solution to A System of Equations
- Graphs of Systems of Equations
- Independent, Inconsistent, and Dependent Systems
- The Method of Solving A System Graphically
Systems of equations
Systems of equations
A collection of two linear equations in two variables is called a
system of linear equations in two variables , or more briefly,
a system of equations . The
pair of equations
is a system of equations. The brace
is used to denote that the two equations occur together (simultaneously).
Solution to a system of equations
Solution to a system
We know that one of the infinitely many solutions to one linear equation in two variables is an ordered pair. An ordered pair that is a solution to both of the equations in a system is called a
solution to the system of equations . For example, the ordered pair
is a solution to the system
since
is a solution to both equations.
Graphs of systems of equations
One method of solving a system of equations is by graphing. We know that the graph of a linear equation in two variables is a straight line. The graph of a system will consist of two straight lines. When two straight lines are graphed, one of three possibilities may result.
Independent, inconsistent, and dependent systems
Independent systems
Systems in which the lines intersect at precisely one point are called
independent systems . In applications, independent systems can arise when the collected data are accurate and complete. For example,
The sum of two numbers is 10 and the product of the two numbers is 21. Find the numbers.
In this application, the data are accurate and complete. The solution is 7 and 3.
Inconsistent systems
Systems in which the lines are parallel are called
inconsistent systems . In applications, inconsistent systems can arise when the collected data are contradictory. For example,
The sum of two even numbers is 30 and the difference of the same two numbers is 0. Find the numbers.
The data are contradictory. There is no solution to this application.
Dependent systems
Systems in which the lines are coincident are called
dependent systems . In applications, dependent systems can arise when the collected data are incomplete. For example.
The difference of two numbers is 9 and twice one number is 18 more than twice the other.
The data are incomplete. There are infinitely many solutions.
The method of solving a system graphically
The method of solving a system graphically
To solve a system of equations graphically: Graph both equations.
- If the lines intersect, the solution is the ordered pair that corresponds to the point of intersection. The system is independent.
- If the lines are parallel, there is no solution. The system is inconsistent.
- If the lines are coincident, there are infinitely many solutions. The system is dependent.
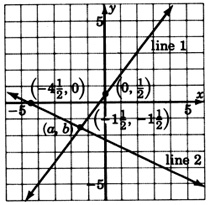
Sample set a
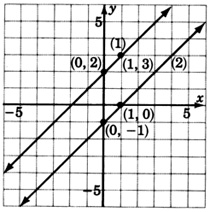
Solve each of the following systems by graphing.
Write each equation in slope-intercept form.
Graph each of these equations.
The lines appear to intersect at the point
. The solution to this system is
, or
Check: Substitute
into each equation.
Got questions? Get instant answers now!
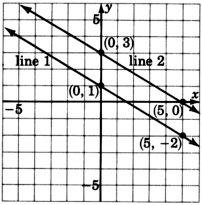
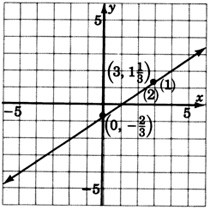
Write each equation in slope-intercept form.
Graph each of these equations.
These lines are parallel. This system has no solution. We denote this fact by writing
inconsistent .
We are sure that these lines are parallel because we notice that they have the same slope,
for both lines. The lines are not coincident because the
-intercepts are different.
Got questions? Get instant answers now!
Write each equation in slope-intercept form.
Both equations are the same. This system has infinitely many solutions. We write
dependent .
Got questions? Get instant answers now!
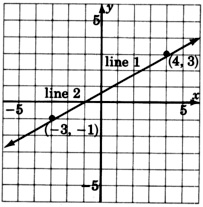
Practice set a
Solve each of the following systems by graphing. Write the ordered pair solution or state that the system is inconsistent, or dependent.
Exercises
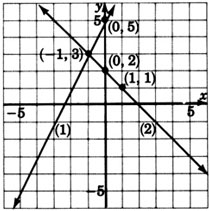
For the following problems, solve the systems by graphing. Write the ordered pair solution, or state that the system is inconsistent or dependent.
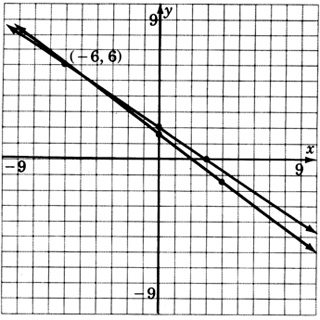
These coordinates are hard to estimate. This problem illustrates that the graphical method is not always the most accurate.
Got questions? Get instant answers now!
Exercises for review