This module is from Elementary Algebra by Denny Burzynski and Wade Ellis, Jr.
Operations with algebraic expressions and numerical evaluations are introduced in this chapter. Coefficients are described rather than merely defined. Special binomial products have both literal and symbolic explanations and since they occur so frequently in mathematics, we have been careful to help the student remember them. In each example problem, the student is "talked" through the symbolic form.Objectives of this module: be able to multiply a polynomial by a monomial, be able to simplify +(a + b) and -(a - b), be able to multiply a polynomial by a polynomial.
Overview
- Multiplying a Polynomial by a Monomial
- Simplifying
and
- Multiplying a Polynomial by a Polynomial
Multiplying a polynomial by a monomial
Multiplying a polynomial by a monomial is a direct application of the distributive property.
Distributive property
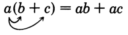
The distributive property suggests the following rule.
Multiplying a polynomial by a monomial
To multiply a polynomial by a monomial, multiply
every term of the polynomial by the monomial and then add the resulting products together.
Sample set a
Practice set a
Determine the following products.
Simplifying
And
And
Oftentimes, we will encounter multiplications of the form
or
These terms will actually appear as
and
Using the distributive property, we can remove the parentheses.
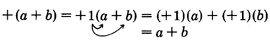
The parentheses have been removed and the sign of each term has remained the same.
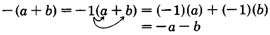
The parentheses have been removed and the sign of each term has been changed to its opposite.
- To remove a set of parentheses preceded by a "
" sign, simply remove the parentheses and leave the sign of each term the same.
- To remove a set of parentheses preceded by a “
” sign, remove the parentheses and change the sign of each term to its opposite sign.
Sample set b
Simplify the expressions.
.
This set of parentheses is preceded by a “
” sign. We can drop the parentheses as long as we change the sign of
every term inside the parentheses to its opposite sign.
Got questions? Get instant answers now!
Practice set b
Simplify by removing the parentheses.
Multiplying a polynomial by a polynomial
Since we can consider an expression enclosed within parentheses as a single quantity, we have, by the distributive property,
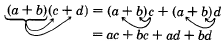
For convenience we will use the commutative property of addition to write this expression so that the first two terms contain
and the second two contain
.
![]()
