| << Chapter < Page | Chapter >> Page > |
When we studied the number line in Section [link] we noted that
Each point on the number line corresponds to a real number, and each real number is located at a unique point on the number line.
![]()
The and signs now have two meanings:
can denote the operation of addition or a positive number.
can denote the operation of subtraction or a negative number.
should be read as "negative eight" rather than "minus eight."
should be read as "four plus negative two" rather than "four plus minus two."
should be read as "negative six plus negative three" rather than "minus six plusminus three."
should be read as "negative fifteen minus negative six" rather than "minus fifteenminus minus six."
should be read as "negative five plus seven" rather than "minus five plus seven."
should be read as "zero minus two."
Write each expression in words.
The opposite of a real number is denoted by placing a negative sign directly in front of the number. Thus, if is any real number, then is its opposite. Notice that the letter is a variable. Thus, need not be positive, and need not be negative.
If is a real number, is opposite on the number line and is opposite on the number line.
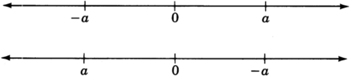
is opposite on the number line. This implies that .
This property of opposites suggests the double-negative property for real numbers.
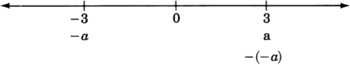
If
, then
and
.
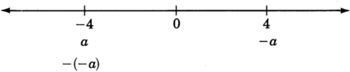
If
, then
and
.
Find the opposite of each real number.
Suppose that is a positive number. What type of number is ?
If is positive, is negative.
Suppose that is a negative number. What type of number is ?
If is negative, is positive.
Suppose we do not know the sign of the number . Can we say that is positive, negative, or that we do notknow ?
We must say that we do not know.
A number is denoted as positive if it is directly preceded by ____________________ .
a plus sign or no sign at all
A number is denoted as negative if it is directly preceded by ____________________ .
For the following problems, how should the real numbers be read ? (Write in words.)
For the following problems, write the expressions in words.
Rewrite the following problems in a simpler form.
( [link] ) There is only one real number for which . What is the number?
0
( [link] ) Simplify .
( [link] ) Simplify .

Notification Switch
Would you like to follow the 'Elementary algebra' conversation and receive update notifications?