6.1 Areas between curves (Page 2/4)

Calculus volume 1 Page 2 / 4

If $R$ is the region bounded by the graphs of the functions $f (x) = \frac{x}{2} + 5$ and $g (x) = x + \frac{1}{2}$ over the interval $[1, 5],$ find the area of region $R .$

$12$ units ²

Got questions? Get instant answers now!

In [link] , we defined the interval of interest as part of the problem statement. Quite often, though, we want to define our interval of interest based on where the graphs of the two functions intersect. This is illustrated in the following example.

Finding the area of a region between two curves 2

If $R$ is the region bounded above by the graph of the function $f (x) = 9 - {(x / 2)}^{2}$ and below by the graph of the function $g (x) = 6 - x,$ find the area of region $R .$

The region is depicted in the following figure.

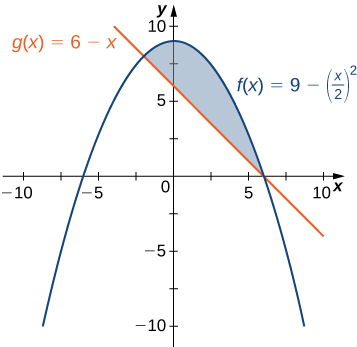
This figure is has two graphs in the first quadrant. They are the functions f(x) = 9-(x/2)^2 and g(x)= 6-x. In between these graphs, an upside down parabola and a line, is a shaded region, bounded above by f(x) and below by g(x). — This graph shows the region below the graph of $f (x)$ and above the graph of $g (x) .$

We first need to compute where the graphs of the functions intersect. Setting $f (x) = g (x),$ we get

\begin{matrix} f (x) & = & g (x) \\ 9 - {(\frac{x}{2})}^{2} & = & 6 - x \\ 9 - \frac{x^{2}}{4} & = & 6 - x \\ 36 - x^{2} & = & 24 - 4 x \\ x^{2} - 4 x - 12 & = & 0 \\ (x - 6) (x + 2) & = & 0. \end{matrix}

The graphs of the functions intersect when $x = 6$ or $x = −2,$ so we want to integrate from $−2$ to $6 .$ Since $f (x) \geq g (x)$ for $−2 \leq x \leq 6,$ we obtain

\begin{matrix} A & = \int_{a}^{b} [f (x) - g (x)] d x \\ = \int_{−2}^{6} [9 - {(\frac{x}{2})}^{2} - (6 - x)] d x = \int_{−2}^{6} [3 - \frac{x^{2}}{4} + x] d x \\ = {[3 x - \frac{x^{3}}{12} + \frac{x^{2}}{2}] |}_{−2}^{6} = \frac{64}{3} . \end{matrix}

The area of the region is $64 / 3$ units ² .

Got questions? Get instant answers now!

If R is the region bounded above by the graph of the function $f (x) = x$ and below by the graph of the function $g (x) = x^{4},$ find the area of region $R .$

$\frac{3}{10}$ unit ²

Got questions? Get instant answers now!

Areas of compound regions

So far, we have required $f (x) \geq g (x)$ over the entire interval of interest, but what if we want to look at regions bounded by the graphs of functions that cross one another? In that case, we modify the process we just developed by using the absolute value function.

Finding the area of a region between curves that cross

Let $f (x)$ and $g (x)$ be continuous functions over an interval $[a, b] .$ Let $R$ denote the region between the graphs of $f (x)$ and $g (x),$ and be bounded on the left and right by the lines $x = a$ and $x = b,$ respectively. Then, the area of $R$ is given by

A = \int_{a}^{b} | f (x) - g (x) | d x .

In practice, applying this theorem requires us to break up the interval $[a, b]$ and evaluate several integrals, depending on which of the function values is greater over a given part of the interval. We study this process in the following example.

Finding the area of a region bounded by functions that cross

If R is the region between the graphs of the functions $f (x) = sin x$ and $g (x) = cos x$ over the interval $[0, π],$ find the area of region $R .$

The region is depicted in the following figure.

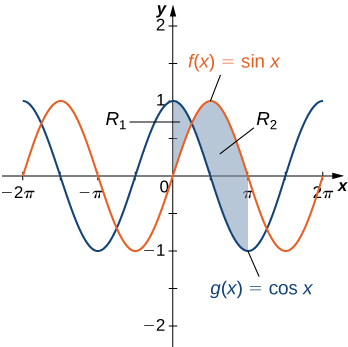
This figure is has two graphs. They are the functions f(x) = sinx and g(x)= cosx. They are both periodic functions that resemble waves. There are two shaded areas between the graphs. The first shaded area is labeled “R1” and has g(x) above f(x). This region begins at the y-axis and stops where the curves intersect. The second region is labeled “R2” and begins at the intersection with f(x) above g(x). The shaded region stops at x=pi. — The region between two curves can be broken into two sub-regions.

The graphs of the functions intersect at $x = π / 4 .$ For $x \in [0, π / 4],$ $cos x \geq sin x,$ so

| f (x) - g (x) | = | sin x - cos x | = cos x - sin x .

On the other hand, for $x \in [π / 4, π],$ $sin x \geq cos x,$ so

| f (x) - g (x) | = | sin x - cos x | = sin x - cos x .

Then

\begin{matrix} A & = \int_{a}^{b} | f (x) - g (x) | d x \\ = \int_{0}^{π} | sin x - cos x | d x = \int_{0}^{π / 4} (cos x - sin x) d x + \int_{π / 4}^{π} (sin x - cos x) d x \\ = {[sin x + cos x] |}_{0}^{π / 4} + {[− cos x - sin x] |}_{π / 4}^{π} \\ = (\sqrt{2} - 1) + (1 + \sqrt{2}) = 2 \sqrt{2} . \end{matrix}

The area of the region is $2 \sqrt{2}$ units ² .

Got questions? Get instant answers now!

If R is the region between the graphs of the functions $f (x) = sin x$ and $g (x) = cos x$ over the interval $[π / 2, 2 π],$ find the area of region $R .$

$2 + 2 \sqrt{2}$ units ²

Got questions? Get instant answers now!

Finding the area of a complex region

Consider the region depicted in [link] . Find the area of $R .$

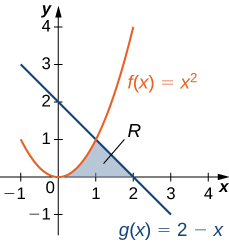
This figure is has two graphs in the first quadrant. They are the functions f(x) = x^2 and g(x)= 2-x. In between these graphs is a shaded region, bounded to the left by f(x) and to the right by g(x). All of which is above the x-axis. The region is labeled R. The shaded area is between x=0 and x=2. — Two integrals are required to calculate the area of this region.

As with [link] , we need to divide the interval into two pieces. The graphs of the functions intersect at $x = 1$ (set $f (x) = g (x)$ and solve for x ), so we evaluate two separate integrals: one over the interval $[0, 1]$ and one over the interval $[1, 2] .$

Over the interval $[0, 1],$ the region is bounded above by $f (x) = x^{2}$ and below by the x -axis, so we have

A_{1} = \int_{0}^{1} x^{2} d x = {\frac{x^{3}}{3} |}_{0}^{1} = \frac{1}{3} .

Over the interval $[1, 2],$ the region is bounded above by $g (x) = 2 - x$ and below by the $x -axis,$ so we have

A_{2} = \int_{1}^{2} (2 - x) d x = {[2 x - \frac{x^{2}}{2}] |}_{1}^{2} = \frac{1}{2} .

Adding these areas together, we obtain

A = A_{1} + A_{2} = \frac{1}{3} + \frac{1}{2} = \frac{5}{6} .

The area of the region is $5 / 6$ units ² .

Got questions? Get instant answers now!

<< Chapter < Page Page > Chapter >>

Read also:

Get Jobilize Job Search Mobile App in your pocket Now!

100% Free Mobile Applications
Receive real-time job alerts and never miss the right job again

Source: OpenStax, Calculus volume 1. OpenStax CNX. Feb 05, 2016 Download for free at http://cnx.org/content/col11964/1.2

Google Play and the Google Play logo are trademarks of Google Inc.

Notification Switch

Would you like to follow the 'Calculus volume 1' conversation and receive update notifications?

Ask

	17 AP Key Terms 17 Endocrine System By OpenStax Start Key Terms
	Biology Final By Anonymous User Start Quiz
	20 AP 20 Blood Vessels Circulation Essay By OpenStax Start Flashcards
	2 Pharmacology Nervous System Essay By Rohini Ajay Start Test
©flickr: Abraham	3 Biology 3 By Sarah Warren Start Test
	3 Enterprise Java Design Patterns By JavaChamp Team Start Quiz
	World Capitals Quiz By Yasser Ibrahim Start Quiz
	2 Arts Society: Theater 2 By Jonathan Long Start Quiz
	2 Lec:2 Randomized Controlled Trials By Janet Forrester Start Quiz
	Anthropology Economic System By Richley Crapo Start Assignment