| << Chapter < Page | Chapter >> Page > |
As gets very large, gets very small. We say that, as increases without bound, approaches 0. As approaches 0, approaches 1. When this happens, the numerator approaches This give us a formula for the sum of an infinite geometric series.
The formula for the sum of an infinite geometric series with is
Given an infinite geometric series, find its sum.
Find the sum, if it exists, for the following:
There is a constant ratio; the series is geometric. and so the sum exists. Substitute and into the formula and simplify to find the sum:
The formula is exponential, so the series is geometric with Find by substituting into the given explicit formula:
Substitute and into the formula, and simplify to find the sum:
Find an equivalent fraction for the repeating decimal
We notice the repeating decimal so we can rewrite the repeating decimal as a sum of terms.
Looking for a pattern, we rewrite the sum, noticing that we see the first term multiplied to 0.1 in the second term, and the second term multiplied to 0.1 in the third term.
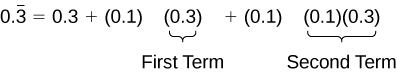
Notice the pattern; we multiply each consecutive term by a common ratio of 0.1 starting with the first term of 0.3. So, substituting into our formula for an infinite geometric sum, we have
Find the sum, if it exists.
At the beginning of the section, we looked at a problem in which a couple invested a set amount of money each month into a college fund for six years. An annuity is an investment in which the purchaser makes a sequence of periodic, equal payments. To find the amount of an annuity, we need to find the sum of all the payments and the interest earned. In the example, the couple invests $50 each month. This is the value of the initial deposit. The account paid 6% annual interest , compounded monthly. To find the interest rate per payment period, we need to divide the 6% annual percentage interest (APR) rate by 12. So the monthly interest rate is 0.5%. We can multiply the amount in the account each month by 100.5% to find the value of the account after interest has been added.
We can find the value of the annuity right after the last deposit by using a geometric series with and After the first deposit, the value of the annuity will be $50. Let us see if we can determine the amount in the college fund and the interest earned.

Notification Switch
Would you like to follow the 'College algebra' conversation and receive update notifications?